How To Find All Real Zeros Of A Function
Roots of Quadratic Equation
The roots of the quadratic equation axtwo + bx + c = 0 are naught but the solutions of the quadratic equation. i.due east., they are the values of the variable (x) which satisfies the equation. The roots of a quadratic role are the x-coordinates of the x-intercepts of the role. Since the degree of a quadratic equation is two, it can have a maximum of two roots. Nosotros tin can discover the roots of quadratic equations using dissimilar methods.
- Factoring (when possible)
- Quadratic Formula
- Completing the Square
- Graphing (used to find just existent roots)
Allow us understand more about the roots of quadratic equation along with discriminant, nature of the roots, the sum of roots, the product of roots, and more than along with some examples.
| 1. | Roots of Quadratic Equation |
| ii. | How to Discover the Roots of Quadratic Equation? |
| three. | Nature of Roots of Quadratic Equation |
| 4. | Sum and Product of Roots of Quadratic Equation |
| 5. | FAQs on Roots of Quadratic Equation |
Roots of Quadratic Equation
The roots of quadratic equation are the values of the variable that satisfy the equation. They are also known equally the "solutions" or "zeros" of the quadratic equation. For instance, the roots of the quadratic equation xtwo - 7x + 10 = 0 are x = 2 and x = 5 because they satisfy the equation. i.e.,
- when x = 2, ii2 - seven(2) + ten = 4 - 14 + 10 = 0.
- when x = 5, 52 - vii(v) + 10 = 25 - 35 + 10 = 0.
But how to find the roots of a general quadratic equation ax2 + bx + c = 0? Let us effort to solve it for x past completing the square.
ax2 + bx = - c
Dividing both sides by 'a',
x2 + (b/a) x = - c/a
Here, the coefficient of x is b/a. One-half of it is b/(2a). Its foursquare is b2/4a2. Adding b2/4a2 on both sides,
10two + (b/a) x + b2/4aii = (b2/4a2) - (c/a)
[ x + (b/2a) ]two = (b2 - 4ac) / 4a2 (using (a + b)² formula)
Taking square root on both sides,
ten + (b/2a) = ±√ (b² - 4ac) / 4a²
x + (b/2a) = ±√ (b² - 4ac) / 2a
Subtracting b/2a from both sides,
ten = (-b/2a) ±√ (b² - 4ac) / 2a (or)
10 = (-b ± √ (b² - 4ac) )/2a
This is known every bit the quadratic formula and information technology can be used to discover any type of roots of a quadratic equation.
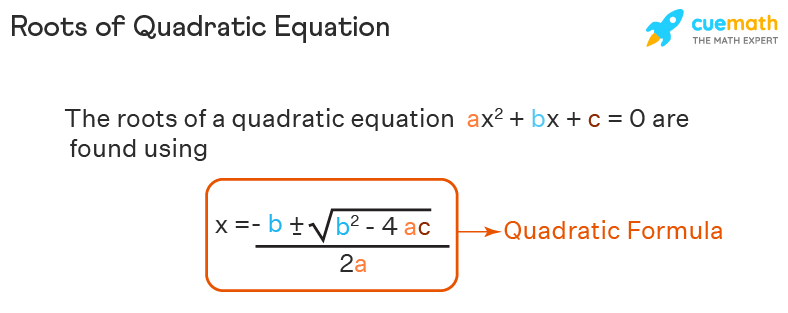
How to Find the Roots of Quadratic Equation?
The process of finding the roots of the quadratic equations is known as "solving quadratic equations". In the previous department, we accept seen that the roots of a quadratic equation can exist found using the quadratic formula. Along with this method, we have several other methods to detect the roots of a quadratic equation. To know about these methods in detail, click hither. Permit united states discuss each of these methods here by solving an example of finding the roots of quadratic equation xtwo - 7x + 10 = 0 (which was mentioned in the previous department) in each case. Note that In each of these methods, the equation should be in the standard grade axtwo + bx + c = 0.
Finding Roots of Quadratic Equation past Factoring
- Factor the left side part.
(ten - two) (x - v) = 0 - Prepare each of these factors to zero and solve.
x - two = 0, ten - 5 = 0
x = ii, x = five.
Finding Roots of Quadratic Equation by Quadratic Formula
- Find a, b, and c values by comparing the given equation with ax2 + bx + c = 0.
Then a = one, b = -7 and c = 10 - Substitute them in the quadratic formula and simplify.
x = [-(-7) ± √((-7)² - 4(1)(ten))] / (two(i))
= [ vii ± √(49 - 40) ] / 2
= [ vii ± √(9) ] / 2
= [ vii ± 3 ] / ii
= (7 + 3) / 2, (7 - 3) / 2
= 10/2, 4/2
= 5, ii
Therefore, x = 2, x = 5.
Finding Roots of Quadratic Equation by Completing Square
- Complete the square on the left side.
(x - (7/2) )two = 9/4 - Solve by taking square root on both sides.
x - 7/two = ± 3/2
x - seven/two = iii/2, x - 7/two = -3/2
x = ten/ii, ten = 4/2
x = 5, x = 2
Finding Roots of Quadratic Equation by Graphing
- Graph the left side office either manually or using the graphing calculator (GDC).
The graph is shown below. - Identify the x-intercepts which are nothing only the roots of the quadratic equation.
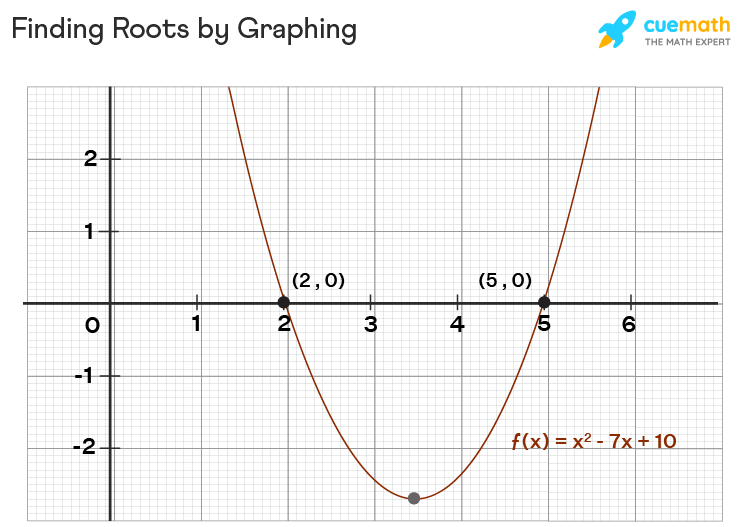
Therefore, the roots of the quadratic equation are ten = 2 and 10 = 5.
We can observe that the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and ten = 5 in each of the methods. Among these methods, the factoring method works only when the quadratic equation is factorable; and we cannot find the complex roots of the quadratic equation using the graphing method. So the best methods that always work for finding the roots are quadratic formula and completing the square methods.
Nature of Roots of Quadratic Equation
The nature of the roots of a quadratic equation talks virtually "how many roots the equation has?" and "what type of roots the equation has?". A quadratic equation tin can take:
- two real and different roots
- 2 complex roots
- two real and equal roots (it means only one real root)
For case, in the higher up example, the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and x = v, where both 2 and 5 are 2 different existent numbers. and so we can say that the equation has two real and different roots. But for finding the nature of the roots, we don't actually need to solve the equation. Nosotros tin can make up one's mind the nature of the roots by using the discriminant. The discriminant of the quadratic equation ax2 + bx + c = 0 is D = b2 - 4ac.
The quadratic formula is x = (-b ± √ (b² - 4ac) )/2a. So this tin be written equally x = (-b ± √ D )/2a. Since the discriminant D is in the square root, we can decide the nature of the roots depending on whether D is positive, negative, or zero.
Nature of Roots When D > 0
Then the above formula becomes,
x = (-b ± √ positive number )/2a
and it gives us two existent and different roots. Thus, the quadratic equation has two real and dissimilar roots when b2 - 4ac > 0.
Nature of Roots When D < 0
And so the in a higher place formula becomes,
x = (-b ± √ negative number )/2a
and it gives the states two complex roots (which are different) as the square root of a negative number is a complex number. Thus, the quadratic equation has 2 complex roots when bii - 4ac < 0.
Note: A quadratic equation tin can never have one complex root. The complex roots always occur in pairs. i.east., if a + bi is a root then a - bi is also a root.
Nature of Roots When D = 0
Then the higher up formula becomes,
ten = (-b ± √ 0)/2a = -b/2a
and hence the equation has only one real root. Thus, the quadratic equation has simply one real root (or two equal roots -b/2a and -b/2a) when b2 - 4ac = 0.
Sum and Production of Roots of Quadratic Equation
Nosotros have seen that the roots of the quadratic equation 102 - 7x + x = 0 are x = 2 and ten = 5. And then the sum of its roots = 2 + 5 = 7 and the product of its roots = two × five = 10. But the sum and the product of roots of a quadratic equation ax2 + bx + c = 0 tin exist institute without really computing the roots. Let us run into how.
Nosotros know that the roots of the quadratic equation axii + bx + c = 0 by quadratic formula are (-b + √ (b² - 4ac) )/2a and (-b - √ (b² - 4ac) )/2a. Allow u.s.a. represent these past x₁ and x₂ respectively.
Sum of Roots of Quadratic Equation
The sum of the roots = x₁ + x₂
= (-b + √ (b² - 4ac) )/2a + (-b - √ (b² - 4ac) )/2a
= -b/2a - b/2a
= -2b/2a
= -b/a
Therefore, the sum of the roots of the quadratic equation ax2 + bx + c = 0 is -b/a.
For the equation, ten2 - 7x + 10 = 0, the sum of the roots = -(-7)/ane = 7 (which was the sum of the actual roots 2 and five).
Production of Roots of Quadratic Equation
The product of the roots = x₁ · ten₂
= (-b + √ (b² - 4ac) )/2a · (-b - √ (b² - 4ac) )/2a
= (-b/2a)2 - (√ b² - 4ac / 2a)ii ( by a² - b² formula)
= b2 / 4a2 - (b2 - 4ac) / 4a2
= bii / 4aii - bii / 4aii + 4ac / 4atwo
= 4ac / 4a2
= c/a
Therefore, the product of the roots of the quadratic equation ax2 + bx + c = 0 is c/a.
For the equation, xii - 7x + ten = 0, the product of the roots = 10/1 = 10 (which was the product of the actual roots 2 and 5).
Important Formulas Related to Roots of Quadratic Equations:
For a quadratic equation ax2 + bx + c = 0,
- The roots are calculated using the formula, x = (-b ± √ (b² - 4ac) )/2a.
- Discriminant is, D = b2 - 4ac.
If D > 0, then the equation has two real and distinct roots.
If D < 0, the equation has two circuitous roots.
If D = 0, the equation has but i real root. - Sum of the roots = -b/a
- Product of the roots = c/a
Topics Related to Roots of Quadratic Equations:
- Roots of Quadratic Equation Figurer
- Roots of Quadratic Equation by Quadratic Formula Calculator
- Roots of Quadratic Equation by Completing Foursquare Calculator
- Roots of Quadratic Equation by Factoring Reckoner
Examples on Roots of Quadratic Equation
go to slidego to slidego to slide

Breakup tough concepts through simple visuals.
Math will no longer be a tough subject, particularly when you understand the concepts through visualizations.
Volume a Free Trial Class
Do Questions on Roots of Quadratic Equation
go to slidego to slide
FAQs on Roots of Quadratic Equation
What are the Roots of a Quadratic Equation?
The roots of a quadratic equation axtwo + bx + c = 0 are the values of the variable (ten) that satisfy the equation. For example, the roots of the equation 102 + 5x + vi = 0 are -two and -3.
How can We Find the Roots of Quadratic Equation?
The roots of a quadratic equation ax2 + bx + c = 0 tin can be found using the quadratic formula that says x = (-b ± √ (b² - 4ac) )/2a. Alternatively, if the quadratic expression is factorable, and so we tin factor it and set the factors to zero to find the roots.
What are Three Types of Roots of Roots of Quadratic Equation?
A quadratic equation ax2 + bx + c = 0 tin have:
- two real and distinct roots when b2 - 4ac > 0.
- two complex roots when b2 - 4ac < 0.
- ii real and equal roots when b2 - 4ac = 0.
How to Determine the Nature of Roots of Quadratic Equation?
The nature of the roots of a quadratic equation axii + bx + c = 0 is determined by its discriminant, D = btwo - 4ac.
- If D > 0, the equation has ii real and distinct roots.
- If D < 0, the equation has 2 complex roots.
- If D = 0, the equation has two equal real roots.
How to Discover the Roots of Quadratic Equation by Completing Square?
To observe the roots of a quadratic equation ax2 + bx + c = 0 by completing square, complete the foursquare on the left side first. Then solve for x by taking the square root on both sides.
How to Find the Roots of Quadratic Equation Using Quadratic Formula?
The quadratic formula says the roots of a quadratic equation axii + bx + c = 0 are given by x = (-b ± √ (b² - 4ac) )/2a. To solve whatever quadratic equation, convert into standard form axii + bx + c = 0, find the values of a, b, and c, substitute them in the quadratic formula and simplify.
Can Both the Roots of Quadratic Equation be Zeros?
Yes, both the roots of a quadratic equation tin be zeros. For example, the two roots of the quadratic equation 10ii = 0 are 0 and 0.
How to Detect the Roots of Quadratic Equation by Factoring?
To discover the roots of a quadratic equation ax2 + bx + c = 0 by factoring, factor its left side function, set each of the factors to zero and solve.
How to Find the Sum and Production of Roots of Quadratic Equation?
For whatsoever quadratic equation ax2 + bx + c = 0,
- the sum of the roots = -b/a
- the product of the roots = c/a
Source: https://www.cuemath.com/algebra/roots-of-quadratic-equation/
Posted by: brownwhipeeir.blogspot.com


0 Response to "How To Find All Real Zeros Of A Function"
Post a Comment