how to find the period of a tangent function

2.three: Graphs of the Tangent and Cotangent Functions

1. Last updated

2. Save as PDF

Page ID

64600

Learning Objectives

• Analyze the graph of \(y=\tan x\).
• Graph variations of \(y=\tan x\).
• Clarify the graph of \(y=\cot x\).
• Graph variations of \(y=\cot x\).

We know the tangent function can be used to observe distances, such as the meridian of a edifice, mountain, or flagpole. But what if we want to measure repeated occurrences of distance? Imagine, for example, a police automobile parked adjacent to a warehouse. The rotating light from the police auto would travel across the wall of the warehouse in regular intervals. If the input is time, the output would be the distance the beam of light travels. The axle of light would repeat the altitude at regular intervals. The tangent function can be used to judge this distance. Asymptotes would exist needed to illustrate the repeated cycles when the beam runs parallel to the wall because, seemingly, the beam of calorie-free could appear to extend forever. The graph of the tangent part would clearly illustrate the repeated intervals. In this section, we will explore the graphs of the tangent and cotangent functions.

Analyzing the Graph of \(y =\tan x\)

We will begin with the graph of the tangent function, plotting points as we did for the sine and cosine functions. Call back that

\[\tan \, ten=\dfrac{\sin \, x}{\cos \, 10}\]

The menstruation of the tangent function is \(\pi\) because the graph repeats itself on intervals of \(k\pi\) where \(yard\) is a abiding. If we graph the tangent function on \(−\frac{\pi}{ii}\) to \(\frac{\pi}{2}\), we can see the behavior of the graph on one complete bike. If we look at any larger interval, we will run across that the characteristics of the graph repeat.

We can determine whether tangent is an odd or fifty-fifty function by using the definition of tangent.

\[\begin{align*} \tan(-x)&= \dfrac{\sin(-x)}{\cos(-x)} \qquad \text{Definition of tangent}\\[4pt] &= \dfrac{-\sin \, x}{\cos \, x} \qquad \text{Sine is an odd role, cosine is even}\\[4pt] &= -\dfrac{\sin \, x}{\cos \, x} \qquad \text{The quotient of an odd and an even function is odd}\\[4pt] &= -\tan \, x \qquad \text{Definition of tangent} \finish{align*}\]

Therefore, tangent is an odd function. We can farther clarify the graphical beliefs of the tangent office past looking at values for some of the special angles, as listed in Table \(\PageIndex{1}\).

Table \(\PageIndex{one}\)

\(x\)	\(−\dfrac{\pi}{two}\)	\(−\dfrac{\pi}{three}\)	\(−\dfrac{\pi}{4}\)	\(−\dfrac{\pi}{6}\)	0	\(\dfrac{\pi}{6}\)	\(\dfrac{\pi}{4}\)	\(\dfrac{\pi}{3}\)	\(\dfrac{\pi}{2}\)
\(\tan x\)	undefined	\(-\sqrt{three}\)	\(–one\)	\(-\dfrac{\sqrt{iii}}{3}\)	0	\(\dfrac{\sqrt{3}}{3}\)	1	\(\sqrt{3}\)	undefined

These points will assist us draw our graph, only we need to determine how the graph behaves where information technology is undefined. If nosotros await more than closely at values when \(\frac{\pi}{3}<ten<\frac{\pi}{2}\), we can use a tabular array to look for a tendency. Because \(\frac{\pi}{three}≈1.05\) and \(\frac{\pi}{2}≈1.57\), nosotros will evaluate \(ten\) at radian measures \(1.05<ten<1.57\) as shown in Table \(\PageIndex{2}\).

Table \(\PageIndex{ii}\)

\(x\)	ane.3	1.5	one.55	1.56
\(\tan x\)	iii.6	fourteen.i	48.ane	92.half-dozen

Equally \(x\) approaches \(\dfrac{\pi}{two}\), the outputs of the role get larger and larger. Because \(y=\tan \, x\) is an odd function, we see the corresponding table of negative values in Table \(\PageIndex{3}\).

Tabular array \(\PageIndex{3}\)

\(ten\)	−one.3	−1.5	−one.55	−1.56
\(\tan x\)	−3.half-dozen	−14.one	−48.1	−92.6

We can see that, as \(x\) approaches \(−\frac{\pi}{ii}\), the outputs get smaller and smaller. Retrieve that there are some values of \(x\) for which \(\cos \, x=0\). For example, \(\cos \left (\frac{\pi}{2} \right)=0\) and \(\cos \left (\frac{iii\pi}{two} \right )=0\). At these values, the tangent role is undefined, and then the graph of \(y=\tan \, 10\) has discontinuities at \(x=\frac{\pi}{2}\) and \(\frac{3\pi}{ii}\). At these values, the graph of the tangent has vertical asymptotes. Figure \(\PageIndex{ane}\) represents the graph of \(y=\tan \, x\). The tangent is positive from \(0\) to \(\frac{\pi}{two}\) and from \(\pi\) to \(\frac{iii\pi}{2}\), corresponding to quadrants I and Three of the unit circumvolve.

Effigy \(\PageIndex{1}\): Graph of the tangent office

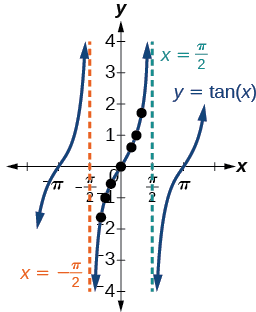
Graphing Variations of \(y = \tan \, x\)

Let'due south alter the tangent curve by introducing vertical and horizontal stretching and shrinking. Equally with the sine and cosine functions, the tangent office can be described by a general equation.

\[y=A\tan(Bx) \nonumber\]

We can identify horizontal and vertical stretches and compressions using values of \(A\) and \(B\). The horizontal stretch can typically be determined from the period of the graph. With tangent graphs, it is often necessary to determine a vertical stretch using a point on the graph.

Because there are no maximum or minimum values of a tangent part, the term amplitude cannot be interpreted as information technology is for the sine and cosine functions. Instead, nosotros will use the phrase stretching/compressing factor when referring to the abiding \(A\).

FEATURES OF THE GRAPH OF \(Y = A \tan(Bx)\)

• The stretching factor is \(|A|\).
• The menstruation is \(P=\dfrac{\pi}{|B|}\).
• The domain is all existent numbers \(x\),where \(ten≠\dfrac{\pi}{2| B |}+\dfrac{π}{| B |}k\) such that \(one thousand\) is an integer.
• The range is \((−\infty,\infty)\).
• To find a pair of asymptotes, solve the equations \(Bx=-\dfrac{\pi}{2}\) and \(Bx=\dfrac{\pi}{2}\). To observe other asymptotes from those use the period of the part.
• \(y=A\tan(Bx)\) is an odd function.

Graphing One Menses of a Stretched or Compressed Tangent Role

We can use what we know about the properties of the tangent function to quickly sketch a graph of any stretched and/or compressed tangent part of the form \(f(x)=A\tan(Bx)\). We focus on a single period of the part including the origin, because the periodic property enables us to extend the graph to the rest of the role'southward domain if we wish. Our limited domain is and then the interval \(\left (−\dfrac{P}{2},\dfrac{P}{2} \right )\) and the graph has vertical asymptotes at \(\pm \dfrac{P}{2}\) where \(P=\dfrac{\pi}{B}\). On \(\left (−\dfrac{\pi}{2},\dfrac{\pi}{2} \right )\), the graph will come up up from the left asymptote at \(x=−\dfrac{\pi}{two}\), cantankerous through the origin, and proceed to increment as it approaches the correct asymptote at \(x=\dfrac{\pi}{2}\). To make the role arroyo the asymptotes at the correct rate, we also demand to set the vertical scale past actually evaluating the role for at least one point that the graph will pass through.

Given the function \(f(x)=A \tan(Bx)\), graph one menstruation.

1. Identify the stretching gene, \(| A |\).
2. Place B and determine the catamenia, \(P=\dfrac{\pi}{| B |}\).
3. To find a pair of asymptotes, solve the equations \(Bx=-\dfrac{\pi}{ii}\) and \(Bx=\dfrac{\pi}{2}\). To find other asymptotes from those utilize the menses of the function.
4. For \(A>0\), the curve increases in betwixt a pair of asymptotes. For \(A<0\) the curve decreases in between a pair of asymptotes.

Case \(\PageIndex{i}\): Sketching a Compressed Tangent

Sketch a graph of i period of the office \(y=0.5\tan \left (\dfrac{\pi}{2}x \correct )\).

Solution

First, we identify \(A\) and \(B\).

Figure \(\PageIndex{2}\)

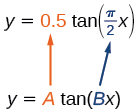
Because \(A=0.five\) and \(B=\dfrac{\pi}{2}\), nosotros can find the stretching/compressing gene and period. The period is \(\dfrac{\pi}{\dfrac{\pi}{two}}=two\). To notice a pair of asymptotes we solve the equations \(\dfrac{\pi}{2}x=-\dfrac{\pi}{2}\) and \(\dfrac{\pi}{ii}10=\dfrac{\pi}{ii}\), which give solutions of \(x=-1\) and \(x=1\), then we know at those ii locations nosotros will take vertical asymptotes. At a quarter menses from the origin, nosotros have

\[\begin{align*} f(0.5)&= 0.5\tan \left (\dfrac{0.5\pi}{two} \right )\\[4pt] &= 0.5\tan \left (\dfrac{\pi}{4} \right )\\[4pt] &= 0.5 \terminate{align*}\]

This means the curve must pass through the points \((0.v,0.5)\), \((0,0)\),and \((−0.5,−0.5)\). The only inflection point is at the origin. Figure \(\PageIndex{3}\) shows the graph of one period of the function.

Figure \(\PageIndex{iii}\)

Exercise \(\PageIndex{one}\)

Sketch a graph of \(f(x)=3\tan \left (\dfrac{\pi}{6}x \correct )\).

Reply

Figure \(\PageIndex{iv}\)

Graphing 1 Period of a Shifted Tangent Function

Now that we can graph a tangent function that is stretched or compressed, we will add a vertical and/or horizontal (or phase) shift. In this instance, we add \(C\) and \(D\) to the general grade of the tangent part.

\[f(x)=A\tan(Bx−C)+D \nonumber\]

The graph of a transformed tangent function is unlike from the basic tangent role \(\tan x\) in several means:

FEATURES OF THE GRAPH OF \(Y = A\tan(Bx−C)+D\)

• The stretching factor is \(| A |\).
• The period is \(\dfrac{\pi}{| B |}\).
• The domain is \(10≠\dfrac{C}{B}+\dfrac{\pi}{| B |}k\),where \(m\) is an integer.
• The range is \((−∞,−| A |]∪[| A |,∞)\).
• To observe a pair of asymptotes, solve the equations \(Bx-C=-\dfrac{\pi}{2}\) and \(Bx-C=\dfrac{\pi}{2}\)
• There is no amplitude.
• The "jerk" point of the curve volition happen on the horizontal line \(y=D\)
• \(y=A \tan(Bx)\) is and odd function because it is the quotient of odd and even functions (sin and cosine respectively).

Howto: Given the part \(y=A\tan(Bx−C)+D\), sketch the graph of ane flow.

1. Express the function given in the form \(y=A\tan(Bx−C)+D\).
2. Identify the stretching/compressing factor, \(| A |\).
3. Identify \(B\) and make up one's mind the period, \(P=\dfrac{\pi}{|B|}\).
4. Solve the equations \(Bx-C=-\dfrac{\pi}{2}\) and \(Bx-C=\dfrac{\pi}{2}\) to observe a pair of asymptotes
5. Plot any three reference points and describe the graph through these points.

Example \(\PageIndex{two}\): Graphing I Period of a Shifted Tangent Function

Graph one period of the office \(y=−2\tan(\pi ten+\pi)−ane\).

Solution

• Step 1. The role is already written in the form \(y=A\tan(Bx−C)+D\).
• Footstep ii.\(A=−2\), so the stretching cistron is \(|A|=2\). Considering of the negative, this bend will exist reflected across the \(ten\)-axis compared to the curve of \(y=tanx\), which means the curve will exist decreasing in betwixt each pair of asymptotes.
• Step iii. \(B=\pi\), then the period is \(P=\dfrac{\pi}{| B |}=\dfrac{\pi}{\pi}=1\).
• Step 4.Solve the equations \(\pi x+\pi=-\dfrac{\pi}{2}\) and \(\pi 10+\pi=\dfrac{\pi}{2}\). For the showtime equation:

\[\begin{align*} \pi ten+\pi&=-\dfrac{\pi}{ii} \\ \pi x&=-\dfrac{\pi}{ii}-\pi \\ \pi x&=-\dfrac{\pi}{two}-\dfrac{2\pi}{2} \\ \pi ten&=-\dfrac{three\pi}{2} \\ x&=-\dfrac{three\pi}{2\pi} \\ 10&=-\dfrac{three}{2} \cease{align*} \]

Similarly, for the second equation nosotros have:

\[\begin{marshal*} \pi 10+\pi&=\dfrac{\pi}{2} \\ \pi ten&=\dfrac{\pi}{two}-\pi \\ \pi x&=\dfrac{\pi}{2}-\dfrac{2\pi}{two} \\ \pi x&=-\dfrac{\pi}{2} \\ ten&=-\dfrac{\pi}{2\pi} \\ x&=-\dfrac{1}{2} \end{align*} \]

• Step 5. We tin can divide the distance of the menstruum by 4 to detect three points in between the asymptotes. Taking one divided past 4 we have \(\dfrac{1}{four}\) or 0.25. Our asymptotes are at -1.five and -0.5. Starting at the left asymptote -one.5 and increasing past 0.25 we land on the values -one.25, -one, and -0.75. Plugging these values in for \(x\) gives us the points \((−1.25,one)\), \((−1,−one)\), and \((−0.75,−3)\). The graph is shown in Figure \(\PageIndex{v}\).

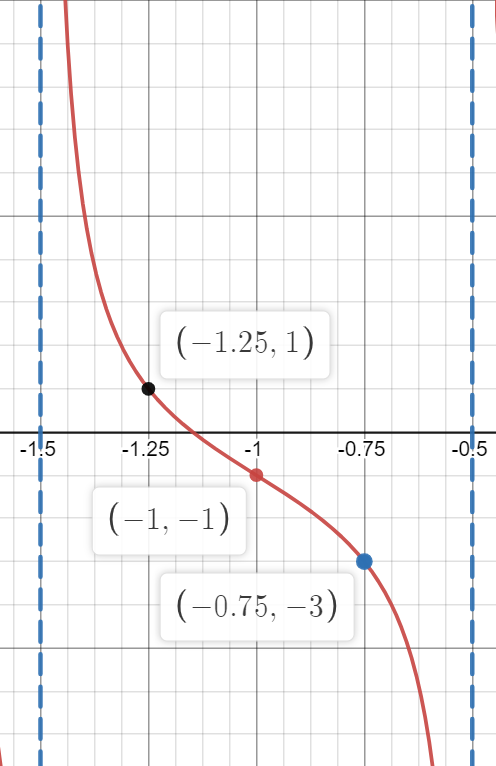
Figure \(\PageIndex{5}\)

Analysis

Annotation that this is a decreasing part considering \(A<0\).

Practice \(\PageIndex{2}\)

How would the graph in Example \(\PageIndex{two}\) wait different if nosotros made \(A=2\) instead of \(−2\)?

Answer

Information technology would be reflected across the line \(y=−1\), becoming an increasing role.

Instance \(\PageIndex{three}\): Identifying the Graph of a Stretched Tangent

Notice a formula for the role graphed in Figure \(\PageIndex{6}\).

Figure \(\PageIndex{six}\): A stretched tangent office

Solution

The graph has the shape of a tangent function.

• Step one. I cycle extends from \(–4\) to \(iv\), and then the menses is \(P=viii\). Since \(P=\dfrac{\pi}{| B |}\), we have \(B=\dfrac{π}{P}=\dfrac{\pi}{8}\).
• Pace two. The equation must have the class \(f(10)=A\tan \left (\dfrac{\pi}{viii}x \right )\).
• Step 3. To find the vertical stretch \(A\),we can utilize the point \((2,two)\). \[\begin{marshal*} two&=A\tan \left (\dfrac{\pi}{8}\cdot 2 \right )\\[4pt] &=A\tan \left (\dfrac{\pi}{four} \correct ) \end{align*}\]

Considering \(\tan \left (\dfrac{\pi}{four} \right )=1\), \(A=2\).

This function would accept a formula \(f(x)=2\tan \left (\dfrac{\pi}{8}10 \right )\).

Practise \(\PageIndex{3}\)

Discover a formula for the office in Figure \(\PageIndex{7}\).

Figure \(\PageIndex{7}\)

Answer

\(g(x)=4\tan(2x)\)

Analyzing the Graph of \(y = \cot 10\)

The terminal trigonometric office we need to explore is cotangent. The cotangent is defined past the reciprocal identity \(cot \, 10=\dfrac{1}{\tan x}\). Detect that the function is undefined when the tangent function is \(0\), leading to a vertical asymptote in the graph at \(0\), \(\pi\), etc. Since the output of the tangent function is all existent numbers, the output of the cotangent function is also all real numbers.

Nosotros can graph \(y=\cot 10\) past observing the graph of the tangent function considering these two functions are reciprocals of one another. See Figure \(\PageIndex{8}\). Where the graph of the tangent function decreases, the graph of the cotangent function increases. Where the graph of the tangent office increases, the graph of the cotangent function decreases.

The cotangent graph has vertical asymptotes at each value of \(x\) where \(\tan x=0\); we show these in the graph below with dashed lines. Since the cotangent is the reciprocal of the tangent, \(\cot ten\) has vertical asymptotes at all values of \(x\) where \(\tan x=0\), and \(\cot x=0\) at all values of \(x\) where \(\tan ten\) has its vertical asymptotes.

Effigy \(\PageIndex{8}\): The cotangent function

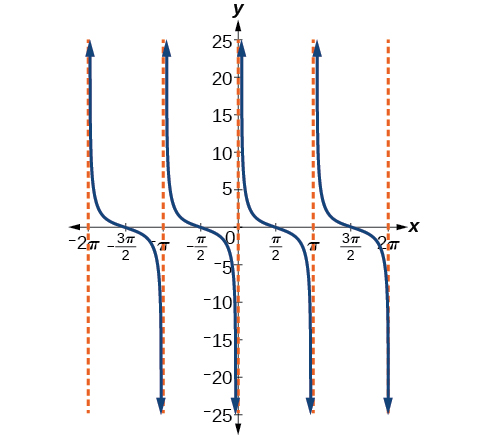
FEATURES OF THE GRAPH OF \(Y = A \cot(BX)\)

• The stretching factor is \(|A|\).
• The period is \(P=\dfrac{\pi}{|B|}\).
• The domain is \(x≠\dfrac{\pi}{|B|}k\), where \(thou\) is an integer.
• The range is \((−∞,∞)\).
• The asymptotes occur at \(ten=\dfrac{\pi}{| B |}k\), where \(k\) is an integer.
• \(y=A\cot(Bx)\) is an odd function.

Graphing Variations of \(y =\cot x\)

We can transform the graph of the cotangent in much the same fashion as we did for the tangent. The equation becomes the following.

\[y=A\cot(Bx−C)+D\]

PROPERTIES OF THE GRAPH OF \(Y = A \cot(Bx-C)+D\)

• The stretching factor is \(| A |\).
• The period is \(\dfrac{\pi}{|B|}\)
• The domain is \(x≠\dfrac{C}{B}+\dfrac{\pi}{| B |}g\),where \(k\) is an integer.
• The range is \((−∞,−|A|]∪[|A|,∞)\).
• A pair of vertical asymptotes occur at the solutions of the equations \(Bx-C=0\) and \(Bx-C=\pi\). To notice others, utilise the distance of the catamenia.
• There is no aamplitude.
• \(y=A\cot(Bx)\) is an odd office considering it is the quotient of even and odd functions (cosine and sine, respectively)

Example \(\PageIndex{four}\): Graphing Variations of the Cotangent Function

Determine the stretching factor, period, and phase shift of \(y=three\cot(4x)\), and then sketch a graph.

Solution

• Step 1.  The stretching factor is \(|A|=3\).
• Stride two. The period is \(P=\dfrac{\pi}{4}\). Dividing this by four gives \(\dfrac{\pi}{16}\). Every time nosotros travel a distance of \(\dfrac{\pi}{xvi}\) we volition be at a vertical asymptote, a wiggle bespeak, or a indicate on either side of the wiggle point.
• Step 3. To find a pair of vertical asymptotes, nosotros solve the equations \(4x=0\) and \(4x=\pi\), which give solutions \(x=0\) and \(x=\dfrac{\pi}{4}\)
• Step four. Plot points in betwixt the vertical asymptotes. Three such points are \(\left (\dfrac{\pi}{xvi},three \correct )\), \(\left (\dfrac{\pi}{eight},0 \right )\) \(\left (\dfrac{three\pi}{16},−three \correct )\).

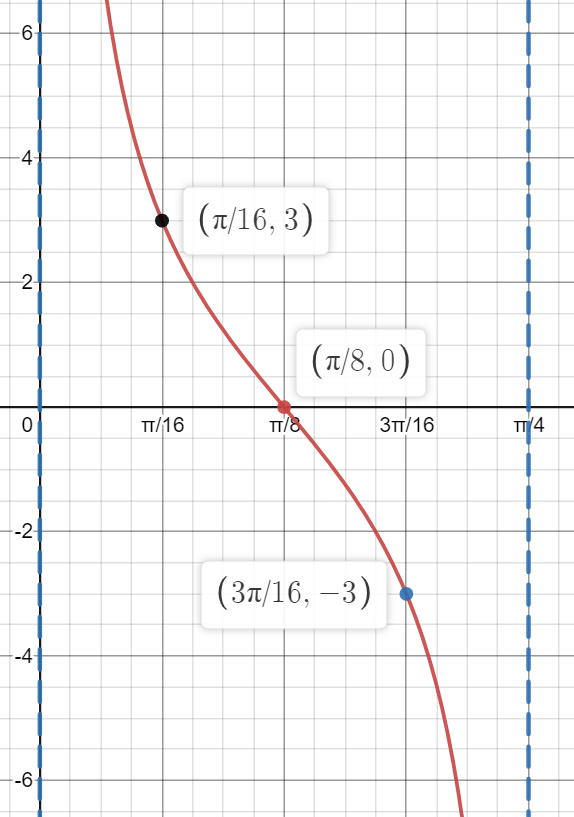
Effigy \(\PageIndex{9}\)

Given a modified cotangent role of the form \(f(x)=A\cot(Bx−C)+D\), graph i catamenia.

1. Identify the stretching factor, \(| A |\). If \(A>0\) the function is decreasing in between a pair of asymptotes. If \(A<0\) so it is increasing.
2. Place the period, \(P=\dfrac{\pi}{|B|}\).
3. Find a pair of asymptotes past solving the equations \(Bx-C=0\) and \(Bx-C=\pi\). To notice others, employ the distance of the flow.
4. Plot whatever three reference points and describe the graph through these points. Points that will be nice to piece of work with are those a distance of a quarter of the menstruation abroad from the asymptotes and in the center of the asymptotes. In the center of the asymptotes is where you should have your wiggle point. The wiggle indicate will be on the line \(y=D\)

Example \(\PageIndex{5}\): Graphing a Modified Cotangent

Sketch a graph of i catamenia of the function \(f(x)=4\cot \left (\dfrac{\pi}{eight}x−\dfrac{\pi}{2} \right )−2\).

Solution

• Step one. \(A=four\),so the stretching cistron is \(4\).
• Step 2. \(B=\dfrac{\pi}{eight}\), and then the flow is \(P=\dfrac{\pi}{| B |}=\dfrac{\pi}{\dfrac{\pi}{8}}=viii\).
• Footstep three.To observe a pair of asymptotes we solve the equations \(\dfrac{\pi}{8}x−\dfrac{\pi}{ii}=0\) and \(\dfrac{\pi}{8}x−\dfrac{\pi}{2}=\pi\). For the showtime equation:

\[ \begin{marshal*} \dfrac{\pi}{eight}x−\dfrac{\pi}{2}&=0 \\ \dfrac{\pi}{8}x&=\dfrac{\pi}{2} \\ x&=\dfrac{\dfrac{\pi}{2}}{\dfrac{\pi}{8}} \\ x&=\dfrac{\pi}{2} \times \dfrac{viii}{\pi} \\ x&=4 \finish{marshal*} \]

For the second equation:

\[ \begin{align*} \dfrac{\pi}{viii}ten−\dfrac{\pi}{2}&=\pi \\ \dfrac{\pi}{8}x&=\pi + \dfrac{\pi}{2} \\ \dfrac{\pi}{eight}x&=\dfrac{ii\pi}{2} + \dfrac{\pi}{2} \\ \dfrac{\pi}{8}10&=\dfrac{three\pi}{2} \\ x&=\dfrac{\dfrac{iii\pi}{ii}}{\dfrac{\pi}{8}} \\ x&=\dfrac{iii\pi}{2} \times \dfrac{8}{\pi} \\ ten&=12 \end{marshal*} \]

The vertical asymptotes are located at \(x=4\) and \(x=12\)

• Step 4. Dividing the period 8 by iv gives 2. Every 2 units nosotros will hit an asymptote, wiggle point, or a betoken on either side of the wiggle signal. The wiggle point will happen one-half mode between the asymptotes on the line \(y=-2\). Starting at the left asymptote and increasing by ii gives the values six, eight, and 10. Three points we can utilise to guide the graph are \((6,ii)\), \((8,−2)\), and \((x,−half dozen)\).

The graph is shown in Figure \(\PageIndex{x}\).

Figure \(\PageIndex{x}\): One menses of a modified cotangent function

Using the Graphs of Trigonometric Functions to Solve Real-Globe Problems

Many real-world scenarios stand for periodic functions and may be modeled by trigonometric functions. Equally an example, let's return to the scenario from the section opener. Have yous ever observed the axle formed by the rotating light on a police motorcar and wondered near the motility of the lite beam itself across the wall? The periodic beliefs of the distance the light shines as a function of fourth dimension is obvious, merely how do we make up one's mind the altitude? We tin can utilise the tangent function.

Example \(\PageIndex{half dozen}\): Using Trigonometric Functions to Solve Existent-World Scenarios

Suppose the function \(y=5\tan(\dfrac{\pi}{4}t)\) marks the distance in the movement of a lite beam from the top of a police car beyond a wall where \(t\) is the time in seconds and \(y\) is the altitude in feet from a point on the wall directly across from the police car.

1. Discover and interpret the stretching factor and period.
2. Graph on the interval \([0,5]\).
3. Evaluate \(f(1)\) and talk over the part's value at that input.

Solution

1. Nosotros know from the general form of \(y=A\tan(Bt)\) that \(| A |\) is the stretching factor and \(\dfrac{\pi}{B}\) is the period.

Figure \(\PageIndex{11}\)

We see that the stretching cistron is \(five\). This means that the beam of lite will have moved \(5\) ft after one-half the period.

The period is \(\dfrac{\pi}{\tfrac{\pi}{four}}=\dfrac{\pi}{1}⋅\dfrac{4}{\pi}=4\). This ways that every \(4\) seconds, the beam of lite sweeps the wall. The distance from the spot across from the police car grows larger as the police automobile approaches.

2. To graph the function, we draw an asymptote at \(t=2\) and use the stretching cistron and catamenia. Encounter Figure \(\PageIndex{12}\)

Effigy \(\PageIndex{12}\)

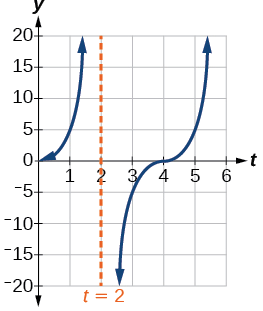
3. period: \(f(i)=v\tan(\frac{\pi}{4}(1))=five(one)=v\); after \(1\) 2nd, the beam of has moved \(five\) ft from the spot across from the police automobile.

Fundamental Equations

Shifted, compressed, and/or stretched tangent part

\(y=A \tan(Bx−C)+D\)

Shifted, compressed, and/or stretched cotangent function

\(y=A \cot(Bx−C)+D\)

Key Concepts

• The tangent part has menstruation \(π\).
• \(f( 10 )=A\tan( Bx−C )+D\) is a tangent with vertical and/or horizontal stretch/compression and shift.
• The cotangent function has period \(\pi\) and vertical asymptotes at \(0,±\pi,±2\pi\),....
• The range of cotangent is \(( −∞,∞ )\), and the function is decreasing at each point in its range.
• The cotangent is zero at \(±\dfrac{\pi}{2},±\dfrac{three\pi}{2}\),....
• \(f(x)=A\cot(Bx−C)+D\) is a cotangent with vertical and/or horizontal stretch/pinch and shift.
• Real-world scenarios can be solved using graphs of trigonometric functions.

Source: https://math.libretexts.org/Courses/Reedley_College/Trigonometry/02:_Graphing_the_Trigonometric_Functions/2.03:_Graphs_of_the_Tangent_and_Cotangent_Functions

Posted by: brownwhipeeir.blogspot.com

Share this post